Capacity Expansion Model v3.3

Modo's forecast generation stack is informed by both known projects and pipelines and an economic capacity expansion model.

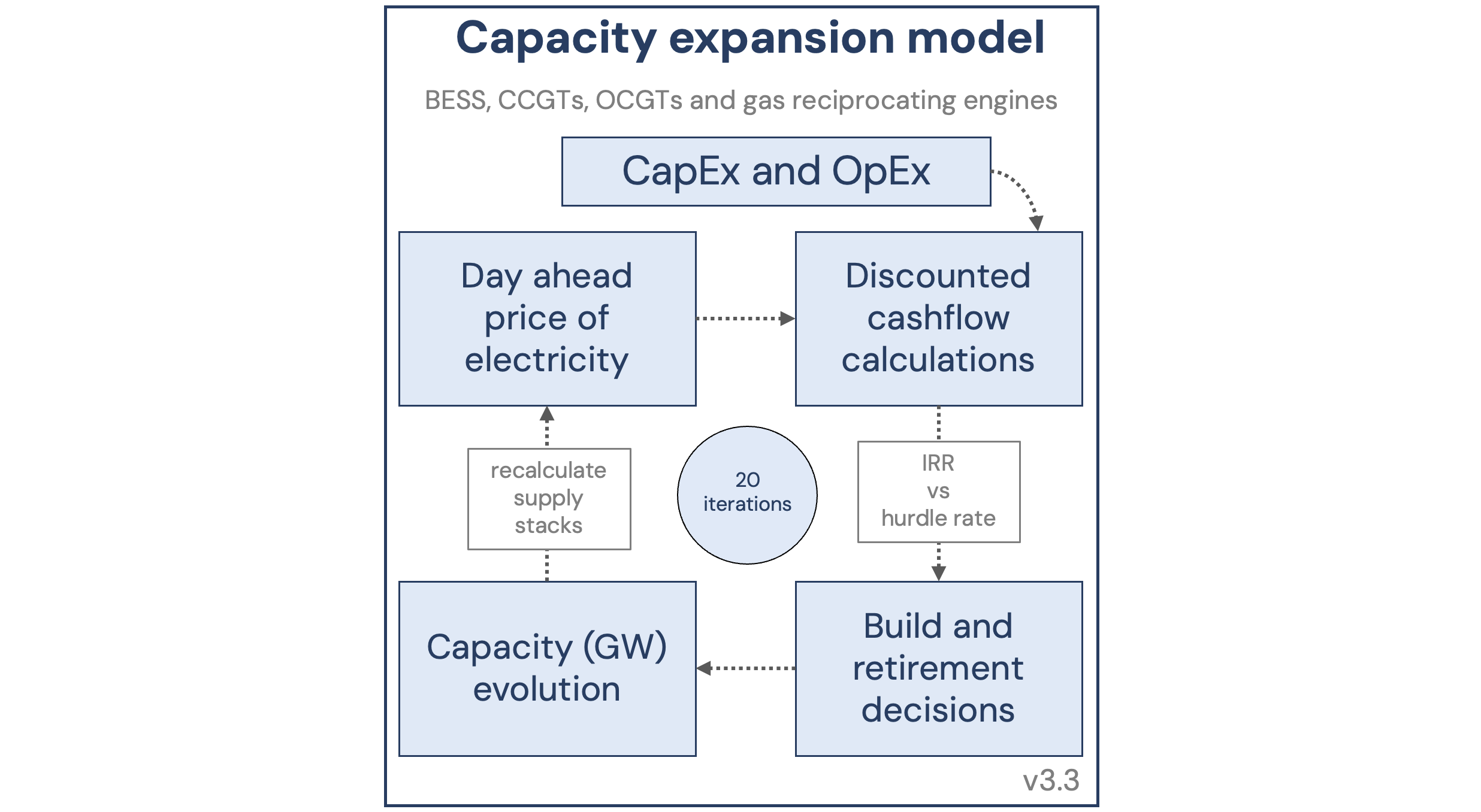
The capacity expansion model represents a feedback loop of capacity market revenues, build-out, and wholesale market revenues for:

• Gas CCGTs
• Gas OCGTs
• Gas reciprocating engines
• Gas CCGTs with Carbon capture and storage (ie abated CCGTs)
• Battery storage (split by duration).

The growth of the capacity of these plants is determined by economics (rather than policy - as opposed to, say, nuclear power).

The Capacity Expansion Model relies on calculating cashflows for individual plants and estimates of CapEx and OpEx for each technology (which change depending on the plant's year of operation). These determine new-build, retirement and retrofit decisions.

More details on how we estimate cashflows are here here. The details on estimates of CaPex and OpEx are here.

Capacities grow (and fall) according to profitability post-2029

The capacity expansion model only dictates the generation stack after 2029 for these types of generators. Before this, the buildout is determined by those plants already in the pipeline. At the end of the forecast horizon, revenues are forward-filled to determine profitability (and buildout) in the mid-2040s onwards.

More details on determining new built, retirement and retrofit decisions can be found here.

It is an iterative model, looping through many cycles to get a stable build-out of generation capacity.

In years that initially have a large number of high power prices (and daily price spreads), gas plant and battery revenues will be high. This triggers more plants to be built. This, in turn, suppresses prices (and spreads), which reduces the cashflows and, therefore, reduces that buildout. By iterating through the capacity expansion model many times and applying some real-world constraints - like how many plants can be built in one year, for example, and how long it takes to build one, we get to our final capacity buildout.

Any under or overbuild of a generation type can be corrected in the next iteration. This results in an iterative process where capacity build-out converges to an optimal solution.